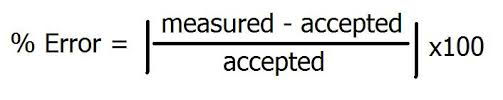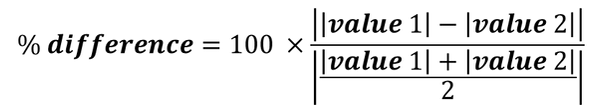AP Physics 1 - We Can Do This!

This lesson is pretty simple and straight forward, but monumentally important.  You don't want to be losing points on the free response section of the AP exam because you didn't take these topics into consideration in an answer that is utterly correct.

​

One of the most complex types of FRQs on the AP Physics 1 Exam is having to explain how you might measure something in the lab and you have to design the experiment yourself.  Uncertainty and Error are important components of being successful at those sorts of questions.

 

Precision- how clustered a set of measured values are or the number of decimal places a measurement goes to.

​

9.284 m is more precise than 9.3 m.

​

A group of values:  1.05 cm, 1.02 cm, and 1.04 cm is precise because the values are closely grouped.  1.05 cm, .94 cm, and 1.88 cm are not precise because they are relatively far apart.  This will lead to percent difference later on.

​

Accuracy - how close a value is to an accepted or standard value.  This is also related to percent error and percent difference.

​

For example, 9.83 m/s/s is very close to the accepted value of 9.81 m/s/s for gravity on earth at sea level.  10.4 m/s/s is not as accurate.

​

Values can be both accurate and precise and that's always your goal in an experiment in physics.

​

Uncertainty has to do with the tool you are using to take the measurement.  A meterstick goes to FOUR decimal places in meters and two decimal places in centimeters.  A spring scale may be in newtons or 1/10th of a newton.

​

Devices for measuring may be digital - electronic balances, voltmeters, range finders, motion detectors or digital thermometers.  Many are analog - they depend on your reading of them.  Metersticks, analog voltmeters, alcohol thermometers, and spring scales are analog.

​

Reading a device adds uncertainty to the measurement.  Even digital devices have uncertainty in that last digit which is why the electronic balances in chemistry would sometimes oscillate between digits.

​

Uncertainty is always a 5 and always one decimal place more precise than the analog device.  You do not add digits to digital readings.  The uncertain digit is already there.

​

For example, in the lab, you measure:  1.3250 m.  The last digit is an estimate - the estimated digit - between the millimeter readings for that position.  It isn't exact.  You are UNCERTAIN about it.  To write this value with uncertainty then:

​

1.3250 +/- .00005 m

​

The original value goes to 4 decimal places.  The uncertainty goes to 5 and you put a 5 there.  Why?  If it's more than 5, the 0 would become a 1.  If it is less than 5, it would stay a zero (like it is).

​

Let's say a spring scale reads in measurements of 0 N, 1 N, 2 N, etc.  There are no divisions between these marks.  If the value falls between the 1 and the 2, you might record the value as 1.1 N, 1.2 N, etc.  Your value has one decimal place.  The uncertainty goes to 2 places and is written as 1.2 +/-.05 N.

​

The more decimal places a device measures to, the more precise it is and the less uncertainty there is in a measurement.

​

Percent Error

​

Percent error in an experiment allows you to demonstrate how close to an expected value (gravity, Planck's constant, pi, etc.) you are.

​

I have always used:  ((O-A)/A) x 100 where O = observed, measured, or lab value and A = accepted or standard value.  When the answer is negative, it's low and when it's positive it's high.  This helps you understand where the error comes from.  In chemistry, your error is usually negative because you spilled or lost material.  In physics, it's often low due to friction.

​

In AP Physics, the College Board wants you to use this equation instead:

​

​

​

​

​

​

​

They chose to use an absolute value because they don't care whether it is (+) or (-). I suggest that you copy this formula onto a sticky note and put it in your lab book on the back of the back page as reference.

​

You will experience 3 types of error in the lab.  Never, ever, ever, explain your error as "human error."

​

1) Experimental - errors or sloppiness in reading a device; faulty equipment (a spring scale that won't zero out, a meter stick with a snubbed end because physics ninjas bang it on the floor repeatedly.

​

2)  Systematic - occurs every time you make a certain measurement.  These are often due to not calibrating or zeroing out a device like a balance.

​

3)  Random - random errors are super irritating.  You can't predict them and often can't find the source.  Changes in temperature, humidity and lighting conditions fit in this category.  The good news is that you should rarely encounter random errors in this class.

​

Percent Difference

​

I feel relatively certain that you've never had to calculate percent difference, but it is easy to do.  Percent difference is used when you don't have an accepted value.  The formula is:  

​

​

​

​

​

​

Value 2 might be the class average or something similar.  Record this on that sticky note for your lab book as well.

​

Significant Digits - a note:

​

Some values are exact.  For example, three trials is exactly 3.  This means that it does not determine the number of significant digits in a calculated value because it's really 3.000000000000000000.

​

Finally, Scientific Rounding:

​

Scientific rounding is used by scientists and technician involved in experimental work.  It allows you to reduce bias in measurements and calculations.

​

For example:  (10.806 N/m)(16.8m) = 181.5408 N.  The 5 is the rounding digit because you only get three significant digits for your answer.

​

Look at the digit you need to round to.  In this case, it is the 1 to the right of the 8 in 181.5408 N.  Even?  Leave it alone and truncate the rest of the value.  Odd?  Round up.

​

In this case, the correct value is 182 N.  This is the same value you would get if you used regular rounding rules.  So let's look at a slightly different value.

​

182.5408 rounded to 3 significant digits.  The rounder is the 5, the rounded value is the 2.  It's even.  Leave it as a 2 and truncate (drop) the rest of the value to 182 N.

​

Why on earth does this matter?

​

When the rounding digits are:

​

1    2     3     4    <---- you always truncate

            5

6     7    8    9     <---- you always round up

​

Scientific rounding of the 5 balances it out and keeps the data from being skewed upward.

​

Now, you should be ready to take the quiz on this section.  This quiz is on Quizizz.com starting mid-July.  You can take it two times.  You may find that it tells you that the quiz has expired when you log into the AP Physics class.  Don't panic.  Just text me on Remind and I'll reset the dates on them.

​

​

 

 

​